
Use substitution to solve the simultaneous equationsġ 3 𝑥 + 2 3 = 𝑦, 6 𝑥 + 3 5 𝑦 = 6 4 5. This confirms that 𝑥 = 2, 𝑦 = 1 is the solution to this system of equations.Įxample 3: Solving Simultaneous Equations by Substitution This is equal to the right-hand side of the equation, so the solution satisfies the second equation. Substituting 𝑥 = 2 and 𝑦 = 1 into the left-hand side of the second equation givesĤ 𝑥 + 3 𝑦 = 4 ( 2 ) + 3 ( 1 ) = 8 + 3 = 1 1. This is equal to the right-hand side of the equation, so the solution satisfies the first equation. Substituting 𝑥 = 2 and 𝑦 = 1 into the left-hand side of the first equation givesĥ 𝑥 − 2 𝑦 = 5 ( 2 ) − 2 ( 1 ) = 1 0 − 2 = 8. We can verify this solution by substituting both values into the two equations to check if they hold true. So, 𝑥 = 2 and 𝑦 = 1 is the solution to this system of equations. We then add 2 to both sides of the equation, gettingįinally, we divide the equation through by 5 to get We can now determine the value of 𝑥 by substituting 𝑦 = 1 into the first ĭividing the equation through by − 2 3 4 yields We now distribute 5 over the parentheses to get Now, we can substitute this expression for 𝑥 into the first equation to construct an equation
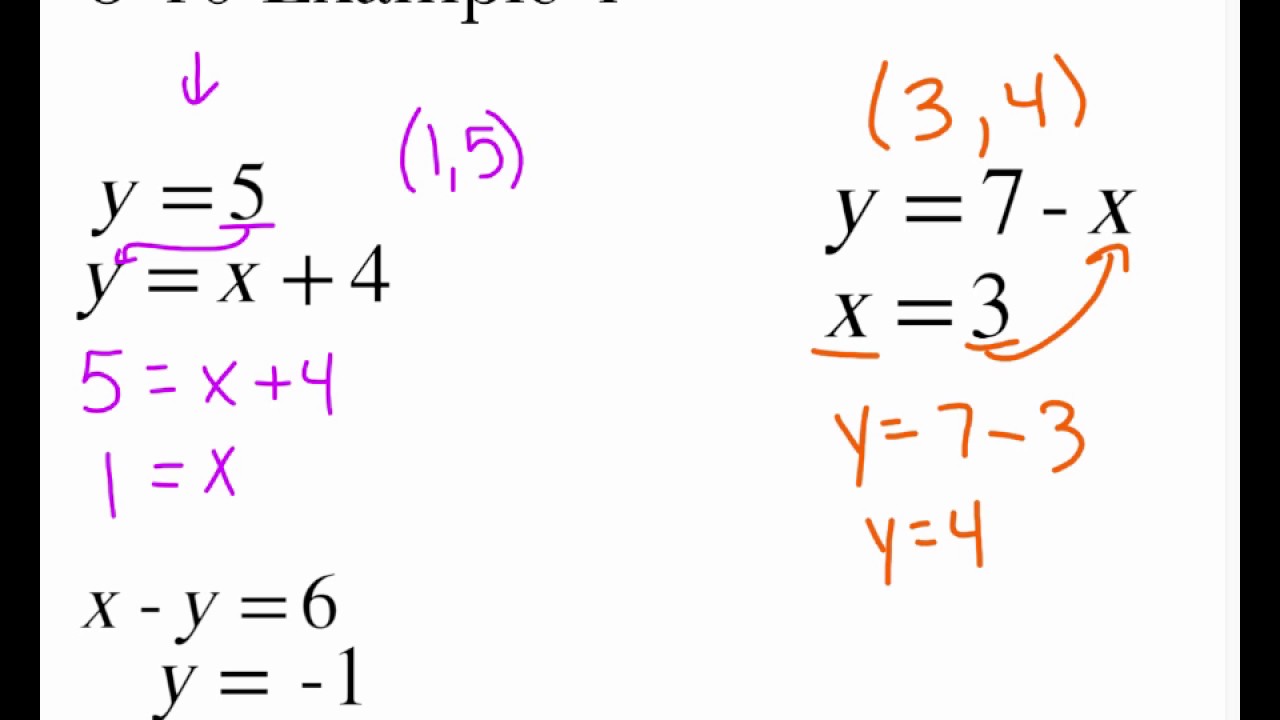
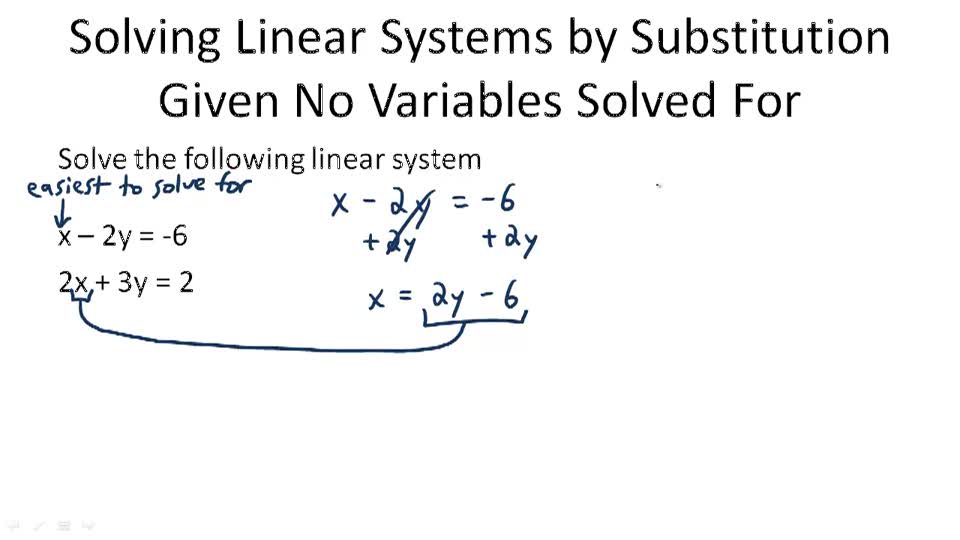
Then, we divide the equation through by 4 to get We subtract 3 𝑦 from both sides of the equation to get None of the equations are already in this form, so we can choose any equation and variable we wish we will We first need to rearrange one of the equations so that a variable is the subject. We are asked to solve a system of two linear equations in two unknowns and we recall that we can do this by using We can generalize this method to attempt to solve any system of two linear equations in two unknowns.Įxample 2: Solving Systems of Linear Equations Using Substitutionĥ 𝑥 − 2 𝑦 = 8, 4 𝑥 + 3 𝑦 = 1 1. In all of these cases, we would arrive at the same solution. It is worth noting that our choice of substitution does not matter we could have rearranged the firstĮquation to make 𝑥 the subject or rearranged the second equation for 𝑥 or This method of solving equations is called substitution, since we substitute a rearrangement of one equation into Since both equations hold true, we have confirmed this is a solution to the Substituting 𝑥 = 1 and 𝑦 = 2 into the left-hand side of the second equation gives Substituting 𝑥 = 1 and 𝑦 = 2 into the left-hand side of the first equation gives Values solve this system of equations by substituting the values into both equations. Therefore, 𝑥 = 1 and 𝑦 = 2 solves the system of equations. Substituting 𝑥 = 1 into the first equation gives We can then substitute this value for 𝑥 into any of these equations to find the value of Must hold true, we can substitute this expression into the other equation to getĭistributing the negative over the parentheses and simplifying yields Since we have now written 𝑦 in terms of 𝑥, and both equations Therefore, if 𝑥 and 𝑦 are solutions to the system of equations, they must also For example, we can rearrange theįirst equation by subtracting 𝑥 from both sides to get Hold true, so we can rearrange one equation to make one variable the subject. To do this, we can note that both equations must Linear equation in either variable, we can solve for that value. Instead, we will use the fact that we can solve any linear equation in one variable.
#Substitution equation systems trial#
WeĬould do this by trial and error however, this will not work for more complicated systems. In other words, we are looking for two values whose sum is 3 and whose difference is − 1.

We want to find a value for 𝑥 and a value for 𝑦 such that both equations hold For example, consider the system of equations
When we are asked to solve a system of equations, this means we are looking for a set of values for the variables
#Substitution equation systems how to#
In this explainer, we will learn how to solve systems of linear equations using substitution.


 0 kommentar(er)
0 kommentar(er)
